
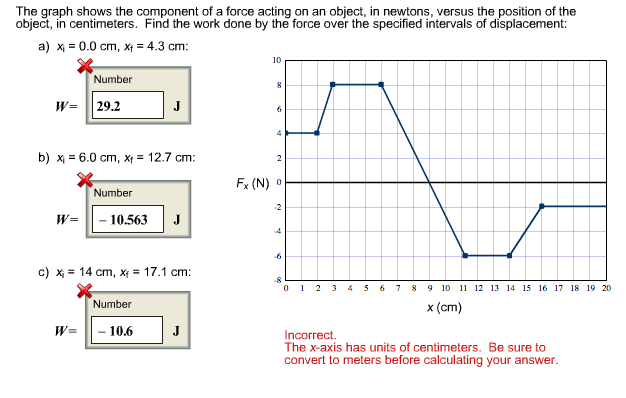
#Workdone by force to change unit vector full#
So statement 2 implies statement 3 ( see full proof).įinally, assume that the third statement is true. The variation of energy for the particle, taking path 1 from A to B and then path 2 backwards from B to A, is 0 thus, the work is the same in path 1 and 2, i.e., the work is independent of the path followed, as long as it goes from A to B.įor example, if a child slides down a frictionless slide, the work done by the gravitational force on the child from the start of the slide to the end is independent of the shape of the slide it only depends on the vertical displacement of the child.Ī force field F, defined everywhere in space (or within a simply-connected volume of space), is called a conservative force or conservative vector field if it meets any of these three equivalent conditions:į → = − ∇ → Φ. For a proof, imagine two paths 1 and 2, both going from point A to point B. The work done by a conservative force is equal to the negative of change in potential energy during that process. This is illustrated in the figure to the right: The work done by the gravitational force on an object depends only on its change in height because the gravitational force is conservative. These and other energy losses are irreversible because of the second law of thermodynamics.Ī direct consequence of the closed path test is that the work done by a conservative force on a particle moving between any two points does not depend on the path taken by the particle. The water drag on a moving boat converts the boat's mechanical energy into not only heat and sound energy, but also wave energy at the edges of its wake. In addition to heat, friction also often produces some sound energy. Usually the energy is turned into heat, for example the heat generated by friction.
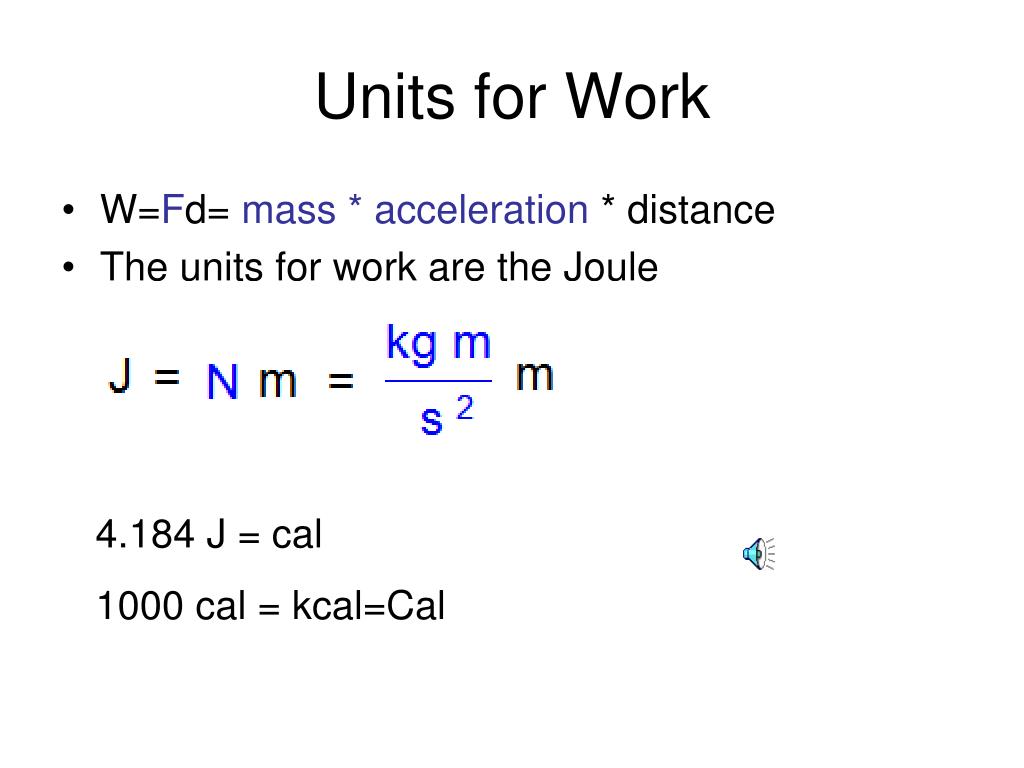
The gravitational force, spring force, magnetic force (according to some definitions, see below) and electric force (at least in a time-independent magnetic field, see Faraday's law of induction for details) are examples of conservative forces, while friction and air drag are classical examples of non-conservative forces.įor non-conservative forces, the mechanical energy that is lost (not conserved) has to go somewhere else, by conservation of energy. Any force that passes the closed path test for all possible closed paths is classified as a conservative force. If the net work done by F at this point is 0, then F passes the closed path test. Though the particle may still be moving, at that instant when it passes point A again, it has traveled a closed path. Then the particle is moved around by other forces, and eventually ends up at A again. Suppose a particle starts at point A, and there is a force F acting on it. Informally, a conservative force can be thought of as a force that conserves mechanical energy. A central force is conservative if and only if it is spherically symmetric. The last two forces are called central forces as they act along the line joining the centres of two charged/magnetized bodies. Other examples of conservative forces are: force in elastic spring, electrostatic force between two electric charges, and magnetic force between two magnetic poles. Gravitational force is an example of a conservative force, while frictional force is an example of a non-conservative force. If the force is not conservative, then defining a scalar potential is not possible, because taking different paths would lead to conflicting potential differences between the start and end points. If a force is conservative, it is possible to assign a numerical value for the potential at any point and conversely, when an object moves from one location to another, the force changes the potential energy of the object by an amount that does not depend on the path taken, contributing to the mechanical energy and the overall conservation of energy. Ī conservative force depends only on the position of the object. Equivalently, if a particle travels in a closed loop, the total work done (the sum of the force acting along the path multiplied by the displacement) by a conservative force is zero. In physics, a conservative force is a force with the property that the total work done in moving a particle between two points is independent of the path taken.


 0 kommentar(er)
0 kommentar(er)
